The continuum component

Normally, this component is responsible for the largest contribution to the ratemeters of each unit in both energy bands: its counts are produced by the diffuse X- and $\gamma$ radiation, cosmic rays and other high energy particles. As already said, the mean count rate usually varies in the range 700-1200 counts/s (sometimes up to 1900-2000 c/s in the 40-700 keV band, especially during the early OPs), and mostly depends on the on-board thresholds, on the Earth magnetic field cut-off rigidity at the BeppoSAX place and on the sky portion facing each detector unit (Earth or whatever X- and $\gamma$-ray sources). This component is characterized by a poissonian statistics, except for a few cases, in which the standard deviation is found to be significantly greater than the square root of the mean counts.

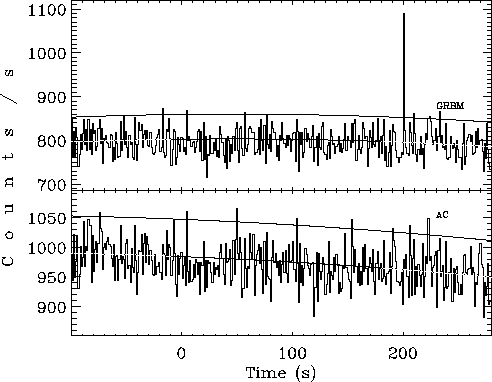
Figure: Example of moving parabolic fit applied to estimate the expected background counts for each bin. For a detailed description, see the section about the late SWTCs. In this case, both the energy bands of unit 1 are shown (OP 04421, May 1998). The +2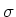$\sigma$ level over the expected background is plotted.

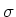The distributions shown in fig.  describe how the counts usually distribute around the correspondent mean values, which are estimated by means of a moving parabolic fit and are used for the GRB quest (see next sections): an example of this moving fit procedure is shown in fig. . The deviations are expressed in terms of $\sigma$, taken as the square root of the expected counts: each distribution, one for each energy band of a given detector unit, has been fitted with a Gaussian.

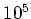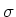Figure: Distributions of the counts (unit 1, GRBM band in the left and AC band in the right panel, respectively) taken from the OP 04421 (May 9-10, 1998), lasted $10^5$ s. A moving parabolic fit estimates the background counts expected for each time bin: here the deviations of the measured counts from the corresponding values are expressed in $\sigma$, calculated as the square root of the expected counts. The 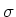$\sigma$s of the best fitting normal distributions are consistent with the poissonian 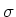$\sigma$s.

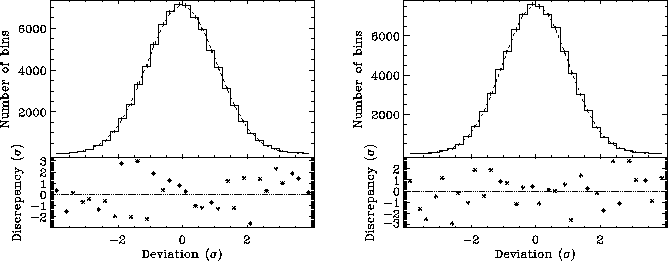While in the case of a typical OP the noise in the count distribution is poissonian ( 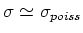$\sigma \simeq \sigma_{poiss}$), there are also some rare OPs, in which another non-poissonian component rises, so that the standard deviation turns out to be significantly greater than the poissonian. The case of OP 00915 shows 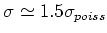$\sigma \simeq 1.5 \sigma_{poiss}$ for unit 1 (fig. ). Nevertheless, this rare property of the counts statistics has been observed only for the softer energy band, i.e. 40-700 keV: the AC band noise always shows a poissonian nature. The situation is summarized in table .

Figure: Distributions of the counts (unit 1, GRBM band in the left and AC band in the right panel, respectively) taken from the OP 00915 (September 13, 1996), lasted 9500 s. This is a rare OP, because it shows a non-poissonian noise, since the standard deviation of its distribution is $\sim 1.5$ times the poissonian $\sigma$.

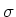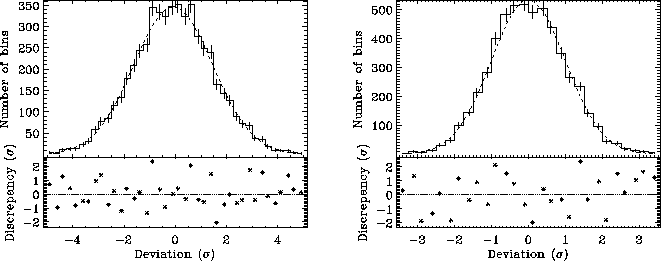Table: Count Distributions over the expected background

OP	Band +	$C_0$	$\sigma$	$\chi^2_r$
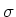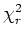	Unit	( $\sigma_{poiss}$)	( $\sigma_{poiss}$)
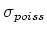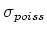04421	GRBM1	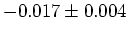$-0.017\pm 0.004$	$1.085\pm 0.003$	0.084
04421	GRBM2	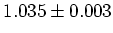$-0.008\pm 0.004$	$1.035\pm 0.003$	0.12
04421	GRBM3	$-0.038\pm 0.004$	$1.045\pm 0.003$	0.14
04421	GRBM4	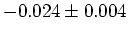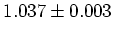$-0.024\pm 0.004$	$1.037\pm 0.003$	0.15
04421	AC1	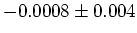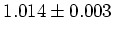$-0.0008\pm 0.004$	$1.014\pm 0.003$	0.077
04421	AC2	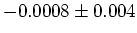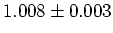$-0.0008\pm 0.004$	$1.008\pm 0.003$	0.015
04421	AC3	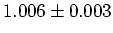$-0.001\pm 0.004$	$1.006\pm 0.003$	0.013
04421	AC4	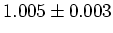$+0.0003\pm 0.004$	$1.005\pm 0.003$	0.011
00915	GRBM1	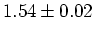$-0.08\pm 0.02$	$1.54\pm 0.02$	0.028
00915	GRBM2	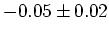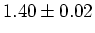$-0.05\pm 0.02$	$1.40\pm 0.02$	0.041
00915	GRBM3	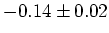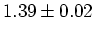$-0.14\pm 0.02$	$1.39\pm 0.02$	0.046
00915	GRBM4	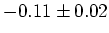$-0.11\pm 0.02$	$1.44\pm 0.02$	0.044
00915	AC1	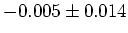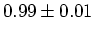$-0.005\pm 0.014$	$0.99\pm 0.01$	0.073
00915	AC2	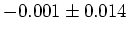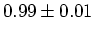$-0.001\pm 0.014$	$0.99\pm 0.01$	0.045
00915	AC3	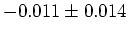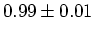$-0.011\pm 0.014$	$0.99\pm 0.01$	0.066
00915	AC4	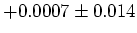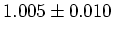$+0.0007\pm 0.014$	$1.005\pm 0.010$	0.034